Here, I will demonstrate how M-theory implies that the string …
Hodge Theory and Gromov-Witten Invariants of Calabi-Yau 3-Folds
I discussed Gromov-Witten Invariants and Hodge integrals on numerous occasions…
4-D Effective String-Theory and No-Singularity Cosmology
All viable quantum cosmological field theories must solve the cosmological …
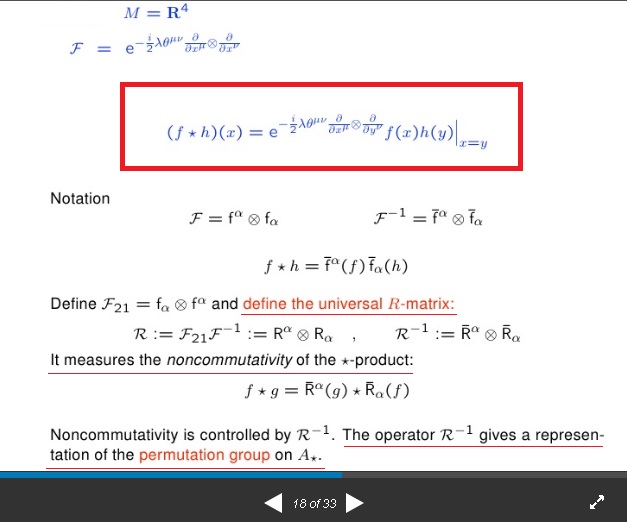
Quantum Geometry, Emergence and Noncommutative Spacetime
I will show that any field theory that supervenes on …
SU(N)-Gauge-Theory from Non-Abelian Nambu-Goldstone Model
All quantum-gravity theories, most famously being String/M-theory and Loop …