Though it is folklore to consider loop quantum gravity as the main rival to M-theory, this is actually far from being correct, and I will show on various occasions why loop quantum gravity fails both, as a quantum gravity theory, and thus, by extension, as a loop quantum cosmology theory as well. Meanwhile, this is a great talk by Andy Strominger and this on LQG. Here, in part 1, I will take a critical look at the Holst action and the Barbero-Immirzi parametric criteria for defining the theory. Before touching on the foundational differences between string-theory (M-theory really) and LQG, let us contrast the actions. Realize, and this is key, that, unlike in LQG, as I showed, the worldsheet quantum theory of string-theory ‘knows’ all about 4-D spacetime physics since in 4-dimensional space-time, the mass of a Dp-brane is:
![Rendered by QuickLaTeX.com \[{T_p}{e^{ - {\Phi _{bos}}}}\prod\limits_{i = 1}^p {\left( {2\pi nR} \right)} \]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-3c2f43a024963feed958e11d0d7d3fbf_l3.png)
and by T-dualizing in the
![]()
direction and factoring the dilaton, the dual is:
![Rendered by QuickLaTeX.com \[\begin{array}{c}({T_p}\left( {2\pi \sqrt {\alpha '} } \right){e^{ - {\Phi _{bos}}}}\prod\limits_{i = 1}^{p - 1} {\left( {2\pi {R_p}} \right)} = \\{T_{p - 1}}{e^{ - \Phi _{bos}^{1/2}}}\prod\limits_{i = 1}^{p - 1} {\left( {2\pi {R_{p - 1}}} \right)} \end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-8810308c79381bf0f22ba417dce0c20b_l3.png)
By matrix world-volume integral reduction on
![Rendered by QuickLaTeX.com \[S_p^D = \, - {T_p}\int\limits_{{\rm{worldvolumes}}} {{d^{p + 1}}} \xi \frac{{\not D_{\mu \nu }^{susy}L}}{{{{\not \partial }_{{v_a}}}}}{e^{ - {\Phi _{bos}}}}{\rm{de}}{{\rm{t}}^{1/2}}G_{ab}^{\exp \left( {H_{p + 1}^{{\rm{array}}}} \right)}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-3ee868f0f83c3f66855aa2539b9a4822_l3.png)
the Polyakov action for the string is hence:
![]()
with ![]() the embedding of the string in target space,
the embedding of the string in target space, ![]() the worldsheet metric, and
the worldsheet metric, and ![]() the spacetime metric. The renormalization Lie-equation for the sigma-model on the string worldsheet entails that
the spacetime metric. The renormalization Lie-equation for the sigma-model on the string worldsheet entails that
![]()
Therefore, the β-function equation gives a definition of the stress-energy tensor for the worldsheet theory:
![]()
with
![]()
By holographic renormalization, it follows that the worldsheet quantum theory ‘knows’ all about spacetime physics
as mathematically attested by
![Rendered by QuickLaTeX.com \[\frac{d}{{{d_{{\sigma _p}}}}}\int\limits_{{\rm{worldvolumes}}}^p {{e^{H_{p + 1}^{{\rm{array}}}}}} + \underbrace {\sum\limits_{{\sigma _p}}^D {{{\left( {S_p^D} \right)}^{ - H_{p + 1}^{{\rm{array}}}}}} }_{{\rm{topologies}}}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-eac6615751f3de7e728719e91821ec83_l3.png)
This is central in comparative analysis with rivals to string-theories, since topologically, none have an analogue to the following sum-term:
![Rendered by QuickLaTeX.com \[\underbrace {\sum\limits_{{\sigma _p}}^D {{{\left( {S_p^D} \right)}^{ - H_{p + 1}^{{\rm{array}}}}}} }_{{\rm{topologies}}}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-b01e5ab7a3152cf7a192573d0a8b576f_l3.png)
where
![]()
would play the role of the renormalization group action for the respective theories, and here are representative rivals. It really boils down to Edward Witten‘s correct claim that all concepts that have theoretical worth, like twistors, noncommutative geometry, spin-foam and what not, can be re-interpreted in, and identified as aspects of, string theory itself and absorbed by it.
This post-series though is about a critical assesment of LGQ. Let me now state the main differentiating factors between LQG and string-theory. What determines whether one is a loop quantum gravity theorist: a non-perturbative canonical quantization of GR, versus a string-theorists, is that the former holds to the view that General Relativity is a correct description of the micro-fundamental degrees of freedom of the gravitational field rather than General Relativity being an effective low energy emergent phenomenon arising at large distances from a more fundamental theory with different degrees of freedom, more in line with the Newtonian-Einsteinian tradition that led to the Standard Model of physics and its associated inflationary cosmology model. Aside, this is an excellent read.
In 4-D spacetime, the general relativistic starting point for canonical loop quantum gravity is given by:
![Rendered by QuickLaTeX.com \[\begin{array}{l}{S_{4{\rm{D}}}}\left[ {e',\omega } \right] = \int_{\tilde M} {\left( {\frac{1}{2}} \right.} {\rm{tr}}\left( {e \wedge e \wedge F} \right)\\\left. { + \frac{1}{\gamma }{\rm{tr}}\left( {e \wedge e \wedge * F} \right)} \right)\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-6ff177d99b80a387dd34aa460f7067ea_l3.png)
with the dynamical variables are the tetrad one-form fields:
![]()
and the ![]() -valued connection
-valued connection ![]() whose curvature is:
whose curvature is:
![]()
Hence, we have the two-form:
![]()
with:
![]()
and ![]() is the Killing form on the Lie algebra
is the Killing form on the Lie algebra ![]() :
:
![]()
with
![]()
the totally antisymmetric tensor given by:
![]()
Now, I can write down the Holst action more informatively:
![Rendered by QuickLaTeX.com \[\begin{array}{*{20}{l}}{{S_{4D}}\left[ {e,\omega } \right] = \int_{{{\tilde M}_4}} {{{\rm{d}}^4}} x{\varepsilon ^{\mu \nu \rho \sigma }}\left( {\frac{1}{2}} \right.{\varepsilon _{IJKL}}}\\{e_\mu ^Ie_\nu ^JF_{\rho \sigma }^{KL}\left. { + \frac{1}{\gamma }e_\mu ^Ie_\nu ^J{F_{\rho \sigma }}_{IJ}} \right)}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-5259c49f9f6455fd1438a77ef93a80e4_l3.png)
In covariant loop quantum gravity, one must work with the time gauge, involving the breaking of the ![]() gauge group into an
gauge group into an ![]() maximal compact subgroup with condition:
maximal compact subgroup with condition:
![]()
Here’s how the Ashtekar-Barbero connection comes in: the phase space must be parametrized by an ![]() -valued connection and its conjugate triad field, and that is exactly the Ashtekar-Barbero connection! Moreover, the compactness of the gauge group ensures that the quantization leads to a mathematically exact kinematical Hilbert space.
-valued connection and its conjugate triad field, and that is exactly the Ashtekar-Barbero connection! Moreover, the compactness of the gauge group ensures that the quantization leads to a mathematically exact kinematical Hilbert space.
- A major question is: since the area and volume operators exhibit discrete spectra, can the Barbero-Immirzi parameter be interpreted as a measure of the area gap in Planck units?
- Is the Ashtekar-Barbero connection metaplectically over-determined?
I will address both questions in upcoming posts.
Back to the Holst action: there are also many connection-constraints-choices after time-gauging. The obvious one is the Lorentz-covariant extension of the Ashtekar-Barbero connection, and crucially, it is commutative with respect to the Dirac-bracket, and thus leads to the same quantum theory as the ![]() formulation in the time gauge. However, to guarantee 4D-diffeomorphicity under quantization, one has another choice, the gauge-shifted connection, hence the Hamiltonian formulation becomes independent of
formulation in the time gauge. However, to guarantee 4D-diffeomorphicity under quantization, one has another choice, the gauge-shifted connection, hence the Hamiltonian formulation becomes independent of ![]() , even at the quantum field level.
, even at the quantum field level.
The main obstacle and the reason why ultimately loop quantum gravity fails is that there provably can be NO group-representation of the associated quantum algebra
Another problem with LQG in 4-dimensions is the gap between the predictions of the quantum theories based on the Ashtekar-Barbero connection and the gauge-shifted connection.
Let us start in 3-D first, and see the interconnections between the gauge-fixing of the Holst action and the Barbero-Immirzi parameter.
One way of tackling the 4-D-gap problem just mentioned is to attempt a dimensional reduction from 4 to 3 via the symmetry group action.
Start from the 4-D Holst action:
![Rendered by QuickLaTeX.com \[\begin{array}{*{20}{l}}{{S_{4D}}\left[ {e,\omega } \right] = \int_{{{\tilde M}_4}} {{{\rm{d}}^4}} x{\varepsilon ^{\mu \nu \rho \sigma }}\left( {\frac{1}{2}} \right.{\varepsilon _{IJKL}}}\\{e_\mu ^Ie_\nu ^JF_{\rho \sigma }^{KL}\left. { + \frac{1}{\gamma }e_\mu ^Ie_\nu ^J{F_{\rho \sigma }}_{IJ}} \right)}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-5259c49f9f6455fd1438a77ef93a80e4_l3.png)
and perform a spacetime compactification while leaving the internal gauge group intact and with topology:
![]()
and:
![]()
the space-like segment with coordinates ![]() , singling out the third spatial component
, singling out the third spatial component ![]() . Now we specify the conditions.
. Now we specify the conditions.
One is a translational invariance along the third spatial direction ![]() ; secondly, that the parallel transport along
; secondly, that the parallel transport along ![]() is geometrically trivial. Thirdly, therefore, it follows that the covariant derivative of the fields along the direction
is geometrically trivial. Thirdly, therefore, it follows that the covariant derivative of the fields along the direction ![]() vanishes. By time-gauging, we then have:
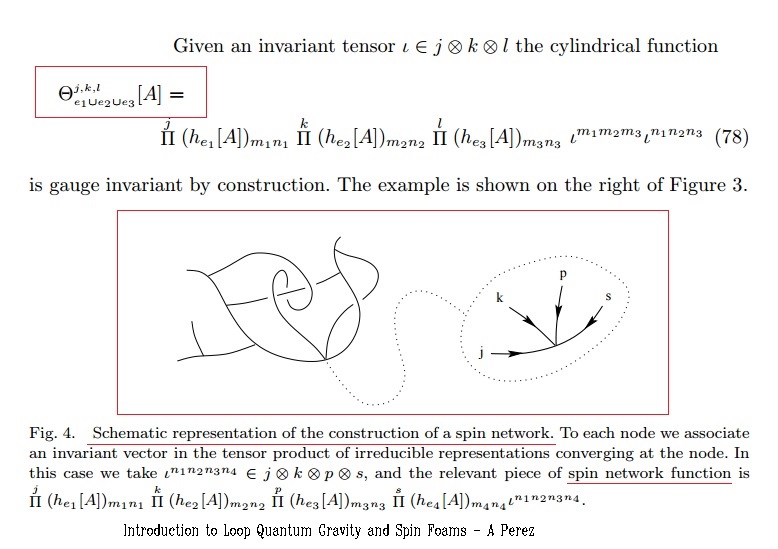
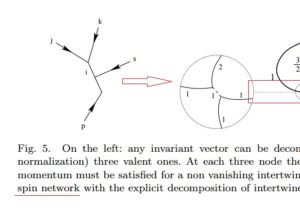
vanishes. By time-gauging, we then have:
![]()
which, given our three conditions, imply that the 4-D Holst action reduces to:
![Rendered by QuickLaTeX.com \[\begin{array}{*{20}{l}}{{S^{{\rm{Red}}}} = - \int_{{S^1}} {\rm{d}} {x^3}\int_{{M_3}} {{{\rm{d}}^3}} x{\varepsilon ^{\mu \nu \rho }}\left( {\frac{1}{2}} \right.}\\{{\varepsilon _{IJKL}}e_3^Ie_\mu ^JF_{\nu \rho }^{KL}\left. { + \frac{1}{\gamma }e_3^Ie_\mu ^J{F_{\nu \rho }}_{IJ}} \right)}\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-22ad282a8a1c8fd7106ab508fa90f4be_l3.png)
with ![]() the three-dimensional spacetime index and
the three-dimensional spacetime index and
![]()
the local volume form on
![]()
With
![]()
One then recovers the total three-dimensional action with the Barbero-Immirzi parameter:
![Rendered by QuickLaTeX.com \[\begin{array}{l}S\left[ {e,x,\omega } \right] = {\int_{{M_3}} {\rm{d}} ^3}x{\varepsilon ^{\mu \nu \rho }}\left( {\frac{1}{2}} \right.{\varepsilon _{IJKL}}\\{x^I}e_\mu ^JF_{\nu \rho }^{KL}\left. { + \frac{1}{\gamma }{x^I}e_\mu ^J{F_{\nu \rho }}_{IJ}} \right)\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-020d8d6de81e54e561046e0ba4e01c91_l3.png)
A major problem for LQG is that the full theory does not imply that the above total three-dimensional action implies three-dimensional gravity, as it ought given compactification and time-gauging. Moreover, the variable ![]() is an additional degree of freedom, and thus must be eliminable. Furthermore, the internal gauge group is not the correct one:
is an additional degree of freedom, and thus must be eliminable. Furthermore, the internal gauge group is not the correct one:
![]()
of Lorentzian three-dimensional gravity, but the wrong one:
![]()
Let me overlook these problems for now. Instead, note that:
![Rendered by QuickLaTeX.com \[\begin{array}{l}S\left[ {e,x,\omega } \right] = {\int_{{M_3}} {\rm{d}} ^3}x{\varepsilon ^{\mu \nu \rho }}\left( {\frac{1}{2}} \right.{\varepsilon _{IJKL}}\\{x^I}e_\mu ^JF_{\nu \rho }^{KL}\left. { + \frac{1}{\gamma }{x^I}e_\mu ^J{F_{\nu \rho }}_{IJ}} \right)\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-020d8d6de81e54e561046e0ba4e01c91_l3.png)
is invariant under the action of
![]()
and admits the infinite-dimensional gauge group:
![]()
as a symmetry group. A member ![]() is hence an
is hence an ![]() -valued function on
-valued function on ![]() and acts on the dynamical variables according to the transformation rules:
and acts on the dynamical variables according to the transformation rules:
![Rendered by QuickLaTeX.com \[\left\{ {\begin{array}{*{20}{c}}{{e_\mu } \to \Lambda \cdot {e_\mu }}\\{x \to \Lambda \cdot x}\\{{\omega _\mu } \to {\rm{A}}{{\rm{d}}_\Lambda }\left( {{\omega _\mu }} \right) - {{\not \partial }_\mu }\Lambda {\Lambda ^{ - 1}}}\end{array}} \right.\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-6950069b637278447538009407a4edb1_l3.png)
with:
![]()
the fundamental action of ![]() on any four-dimensional vector
on any four-dimensional vector ![]() and:
and:
![]()
the adjoint action of
![]()
on any Lie algebra element
![]()
and our theory is invariant under spacetime diffeomorphisms, as it ought to be for General Relativity. Infinitesimal diffeomorphisms are generated by vector fields
![]()
Thus, their action on the dynamical variables is given by the Lie derivatives:
![Rendered by QuickLaTeX.com \[\left\{ {\begin{array}{*{20}{c}}{e \to {L^{lie}}_\upsilon e}\\{x \to {L^{lie}}_\upsilon x = {\nu ^\mu }{{\not \partial }_\mu }x}\\{\omega \to {L^{lie}}_\upsilon \omega }\end{array}} \right.\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-4eb39924be60612865ab8bf5a634cc02_l3.png)
where for any one-form ![]() , we have:
, we have:
![]()
The symmetries mentioned above are expected of a theory of gravity in first order variables formulation. However, in 3-D, such symmetries alone imply a collapse to ![]() BF theory, which is not isomorphic to the model above, and problematically, this would imply that our Lagrangian admits additional symmetries, as can be seen by the fact that
BF theory, which is not isomorphic to the model above, and problematically, this would imply that our Lagrangian admits additional symmetries, as can be seen by the fact that
![Rendered by QuickLaTeX.com \[\begin{array}{l}S\left[ {e,x,\omega } \right] = {\int_{{M_3}} {\rm{d}} ^3}x{\varepsilon ^{\mu \nu \rho }}\left( {\frac{1}{2}} \right.{\varepsilon _{IJKL}}\\{x^I}e_\mu ^JF_{\nu \rho }^{KL}\left. { + \frac{1}{\gamma }{x^I}e_\mu ^J{F_{\nu \rho }}_{IJ}} \right)\end{array}\]](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-020d8d6de81e54e561046e0ba4e01c91_l3.png)
is invariant under rescaling symmetry and translational symmetry. This destroys the time gauge accessibility of the theory and any 4-D equivalence.
So, in this post, part 1, we have made progress with the spacetime compactification and the 3-D Holst-reduction yet we have hit some serious problems, which will be the topic of the next few posts.


1 Response
Loop Quantum Gravity: the Barbero-Immirzi Parameter
Tuesday, October 11, 2016[…] I showed that in 4-D spacetime, the general relativistic starting point for canonical loop quantum gravity is given by: […]