Toroidal compactifications of 11-D supergravity naturally induce ![]() exceptional symmetries in
exceptional symmetries in ![]() that can be realized as U-duality symmetries of M-theory upon Z-discretization and without Betti-truncations. Hence, exceptional field theory based on the modular group
that can be realized as U-duality symmetries of M-theory upon Z-discretization and without Betti-truncations. Hence, exceptional field theory based on the modular group ![]() uses a dimensionally extended spacetime to 12-D that fully covariantizes supergravity under the U-duality symmetry groups of M-theory. By mirror symmetry, there ought to be a deep internal symmetry induced between M-theory and F-theory upon KK-reduction to Type-IIB SUGRA. In the
uses a dimensionally extended spacetime to 12-D that fully covariantizes supergravity under the U-duality symmetry groups of M-theory. By mirror symmetry, there ought to be a deep internal symmetry induced between M-theory and F-theory upon KK-reduction to Type-IIB SUGRA. In the ![]() formalism taking the 6-8 limit, the content of the theory is given by the action:
formalism taking the 6-8 limit, the content of the theory is given by the action:
![]()
with:
![]()
and:
![]()
where the Chern-Simons-topological Lagrangian has covariant variational form:
![]()
with:
![]()
![]()
and the Yang-Mills field equation for the covariant field strength form ![]() is:
is:
![]()
Thus, we can derive the Chern-Simons-type topological action:
![]()
with:
![]()
and:
![]()
and the covariant curvature form ![]() and holomorphic curvature form
and holomorphic curvature form ![]() are, respectively:
are, respectively:
![]()
and:
![]()
where the Ramond-Ramond gauge-coupling sector is given by the action:
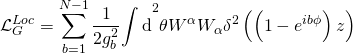
and the Ramond-Ramond term being:
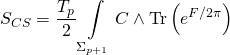
thus giving us the Type-IIB Calabi-Yau three-fold superpotential:
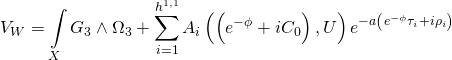
Before we can see the duality relations between M-theory and F-theory elliptic fibrational Standard-Model constructions, note that the topologically mixed Yang-Mills action:
![]()
where the corresponding Chern-Simons action is:
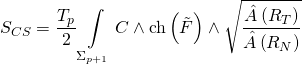
with the Ramond-Ramond coupling-term:
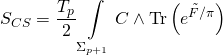
has variational action:
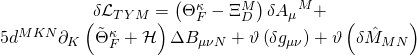
with:
![]()
![]()
![]()
![]()
Now, since 11-D SUGRA on a torus is equivalent to Type-IIB string-theory on a circle, the action of the modular group on the Type-IIB axio-dilaton allows us to take the zero limit of:
![]()
and by mirror symmetry, we get a Type-IIA dimensional uplift to M-theory, given that in the Einstein frame, the Type-IIB bosonic SUGRA action is:
![]()
with:
![]()
![]()
![]()
This is one aspect of appreciating, via the exceptional field theory (EFT) modular group action, the F-theory/M-theory duality. The essence of EFT is thus a deeper double duality relating M-theory/Type-IIA and F-theory/Type-IIB. Key is the role of U-duality in the modular holomorphic action on the Neveu-Schwarz sector of Type-IIB. Our generalized diffeomorphisms, generated by a vector ![]() , act fully locally on
, act fully locally on ![]() yielding the Lie derivative
yielding the Lie derivative ![]() that differs from the classic Lie derivative
that differs from the classic Lie derivative ![]() by a Calabi-Yau induced
by a Calabi-Yau induced ![]() -tensor and is implicitly defined by the transformation rules for a generalized vector:
-tensor and is implicitly defined by the transformation rules for a generalized vector:
![]()
![]()
The associated diffeomorphism algebra has an exceptional field bracket:
![]()
with closure condition:
![]()
The action diffeomorphism-symmetries are parametrized by vector bundles over the metaplectic space and take the form:
![]()
with:
![]()
where the gauge vector transforms as:
![]()
The corresponding generalized exceptional scalar metric ![]() has the following property:
has the following property:
![]()
which decomposes in light of the orbifold blow-up:
![]()
as:
![]()
thus allowing us to define the crucial exceptional metric:
![]()
Since the full Type-IIB Calabi-Yau superpotential is given by:
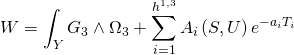
where the Kähler Type-IIB orientifold moduli is:
![]()
with:
![]()
and the volume of the divisor, ![]() , is:
, is:
![]()
with:
![]()
thus we now have the ingredients to write the modular exceptional field theory action as:
![]()
with the exceptional Ricci scalar:
![]()
the kinetic part:
![]()
and the gauge term:
![]()
and the 10+3-D Chern-Simons topological term:
![Rendered by QuickLaTeX.com \displaystyle \begin{array}{l}{{\mathcal{L}}_{{top}}}=\kappa \int{{{{d}^{{10}}}}}x{{d}^{3}}Y{{\varepsilon }^{{{{\mu }_{1}}...{{\mu }_{{10}}}}}}\frac{1}{4}{{\varepsilon }_{{\alpha \beta }}}{{\varepsilon }_{{\gamma \delta }}}\left[ {\frac{1}{5}} \right.{{\partial }_{s}}{{\Omega }_{{{{\mu }_{1}}...{{\mu }_{5}}}}}^{{\alpha \beta ss}}\\{{\Omega }_{{{{\mu }_{6}}...{{\mu }_{{10}}}}}}^{{\gamma \delta ss}}-\frac{5}{2}{{\Gamma }_{{{{\mu }_{1}}{{\mu }_{2}}}}}^{s}{{J}_{{{{\mu }_{3}}...{{\mu }_{6}}}}}^{{\alpha \beta s}}{{J}_{{{{\mu }_{7}}...{{\mu }_{{10}}}}}}^{{\gamma \delta }}\\+\frac{{10}}{3}2{{\Omega }_{{{{\mu }_{1}}...{{\mu }_{3}}}}}^{{\alpha s}}{{\Omega }_{{{{\mu }_{4}}...{{\mu }_{6}}}}}^{{\beta s}}\left. {{{J}_{{{{\mu }_{7}}...{{\mu }_{{10}}}}}}^{{\gamma \delta }}} \right]\end{array}](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-c40521079942e78090ce506ff0858fb8_l3.png)
where the potential has the form:
![Rendered by QuickLaTeX.com \displaystyle \begin{array}{l}V=\frac{1}{4}{{\wp }^{{ss}}}\left( {{{\partial }_{s}}{{\Omega }^{{\alpha \beta }}}{{\partial }_{s}}{{\Omega }_{{\alpha \beta }}}+{{\partial }_{s}}{{g}^{{\mu \nu }}}{{\partial }_{s}}{{g}_{{\mu \nu }}}+{{\partial }_{s}}\text{In}g{{\partial }_{s}}\text{In}g} \right)\\+\frac{9}{{32}}{{\wp }^{{ss}}}{{\partial }_{s}}\text{In}{{\wp }_{{ss}}}{{\partial }_{s}}\text{In}{{\wp }_{{ss}}}-\frac{1}{2}{{\wp }^{{ss}}}{{\partial }_{s}}\text{In}{{\wp }_{{ss}}}\text{In}g\\+\wp _{{ss}}^{{3/4}}\left[ {\frac{1}{4}} \right.{{\Omega }^{{\alpha \beta }}}{{\partial }_{\alpha }}{{\Omega }^{{\gamma \delta }}}{{\partial }_{\beta }}{{\Omega }_{{\gamma \delta }}}+\frac{1}{2}{{\Omega }^{{\alpha \beta }}}{{\partial }_{\alpha }}{{\Omega }^{{\gamma \delta }}}{{\partial }_{\gamma }}{{\Omega }_{{\delta \beta }}}\\+{{\partial }_{\alpha }}{{\Omega }^{{\alpha \beta }}}{{\partial }_{\beta }}\text{In}\left( {{{g}^{{1/2}}}\wp _{{ss}}^{{3/4}}} \right)+\\\frac{1}{4}{{\Omega }^{{\alpha \beta }}}\left( {{{\partial }_{\alpha }}} \right.{{g}^{{\mu \nu }}}{{\partial }_{\beta }}{{g}_{{\mu \nu }}}+{{\partial }_{\alpha }}\text{Ing}{{\partial }_{\beta }}\text{In}g+\frac{1}{4}{{\partial }_{\alpha }}\text{In}{{\wp }_{{ss}}}{{\partial }_{\beta }}\text{In}{{\wp }_{{ss}}}\\+\left. {\frac{1}{2}\left. {{{\partial }_{\alpha }}\text{In}g{{\partial }_{\beta }}\text{In}{{\wp }_{{ss}}}} \right)} \right]\end{array}](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-11e43105f09fb46e9bcbc0863bfedc10_l3.png)
This is a theory dynamically equivalent to 11-D SUGRA and Type-IIB under covariantized U-duality group-action. However, the gauged kinetic terms ![]() corresponding to the gauge form
corresponding to the gauge form ![]() appears only topologically in:
appears only topologically in:
![Rendered by QuickLaTeX.com \displaystyle \begin{array}{*{20}{l}} {{{\mathcal{L}}_{{top}}}=\kappa \int{{{{d}^{{10}}}}}x{{d}^{3}}Y{{\varepsilon }^{{{{\mu }_{1}}...{{\mu }_{{10}}}}}}\frac{1}{4}{{\varepsilon }_{{\alpha \beta }}}{{\varepsilon }_{{\gamma \delta }}}\left[ {\frac{1}{5}} \right.{{\partial }_{s}}{{\Omega }_{{{{\mu }_{1}}...{{\mu }_{5}}}}}^{{\alpha \beta ss}}} \\ {{{\Omega }_{{{{\mu }_{6}}...{{\mu }_{{10}}}}}}^{{\gamma \delta ss}}-\frac{5}{2}{{\Gamma }_{{{{\mu }_{1}}{{\mu }_{2}}}}}^{s}{{J}_{{{{\mu }_{3}}...{{\mu }_{6}}}}}^{{\alpha \beta s}}{{J}_{{{{\mu }_{7}}...{{\mu }_{{10}}}}}}^{{\gamma \delta }}} \\ {+\frac{{10}}{3}2{{\Omega }_{{{{\mu }_{1}}...{{\mu }_{3}}}}}^{{\alpha s}}{{\Omega }_{{{{\mu }_{4}}...{{\mu }_{6}}}}}^{{\beta s}}\left. {{{J}_{{{{\mu }_{7}}...{{\mu }_{{10}}}}}}^{{\gamma \delta }}} \right]} \end{array}](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-af47b800b5a7cb95241241c56fa9d29c_l3.png)
Hence, the EoM for the field ![]() is given by:
is given by:
![]()
Since exceptional field theory based on the modular group ![]() uses a dimensionally extended spacetime to 12-D that fully covariantizes supergravity under the U-duality symmetry groups of M-theory, homological mirror symmetry entails there ought to be a deep internal symmetry induced between M-theory and F-theory upon dimensional-reduction to Type-IIB SUGRA which in the
uses a dimensionally extended spacetime to 12-D that fully covariantizes supergravity under the U-duality symmetry groups of M-theory, homological mirror symmetry entails there ought to be a deep internal symmetry induced between M-theory and F-theory upon dimensional-reduction to Type-IIB SUGRA which in the ![]() formalism, taking the 6/8 Klebanov-Witten limit, is defined by the action:
formalism, taking the 6/8 Klebanov-Witten limit, is defined by the action:
![]()
We are now in a position to explore this EFT-duality between M-theory and F-theory, noting that it is a duality that is rich in F-theory phenomenology, and by the Type-IIB duality, such phenomenology is inherited by 11-D SUGRA under U-duality. ![]() -EFT is equivalent to both, 11-D SUGRA and Type-IIB SUGRA. The field content for EFT consists of:
-EFT is equivalent to both, 11-D SUGRA and Type-IIB SUGRA. The field content for EFT consists of:
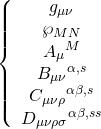
the field content for M-theory consists of:
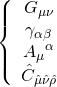
and that of Type-IIB being:
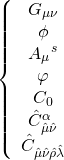
The Kaluza-Klein and gauge fields for M-theory are, respectively:
![]()
![]()
![]()
and:
![]()
![]()
![]()
For the split-Type-IIB theory, the Kaluza-Klein and gauge fields are respectively:
![]()
![]()
![]()
where we have:
![]()
We then parametrize ![]() in terms of the familiar axio-dilaton
in terms of the familiar axio-dilaton ![]() as:
as:
![]()
The ![]() -EFT/11-D/Type-IIB duality can now be written in terms of the gauge field equations as:
-EFT/11-D/Type-IIB duality can now be written in terms of the gauge field equations as:
![]()
![]()
![Rendered by QuickLaTeX.com \displaystyle \begin{array}{l}{{C}_{{\mu \nu \rho }}}^{{\alpha \beta ,s}}={{\varepsilon }^{{\alpha \beta }}}{{{\hat{C}}}_{{\mu \nu \rho s}}}+3{{{\hat{C}}}_{{\left[ {\left. \mu \right|\left. s \right|} \right.}}}^{{\left[ \alpha \right.}}{{C}_{{\left. {\nu \rho } \right]}}}^{{\left. \beta \right]}}\\-2{{{\hat{C}}}_{{\left[ {\left. \mu \right|s} \right.}}}^{\alpha }{{{\hat{C}}}_{{\left. \nu \right|\left. s \right|}}}^{\beta }{{A}_{{\left. \rho \right]}}}^{s}\end{array}](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-252ac42ab1bbdde2c8c3bb805ae56ae3_l3.png)
![Rendered by QuickLaTeX.com \displaystyle \begin{array}{l}{{D}_{{\mu \nu \rho \sigma }}}^{{\alpha \beta ,ss}}={{\varepsilon }^{{\alpha \beta }}}\left( {{{{\hat{C}}}_{{\mu \nu \rho \sigma }}}+4{{A}_{{\left[ \mu \right.}}}^{s}{{{\hat{C}}}_{{\left. {\nu \rho \sigma } \right]s}}}} \right)\\+6{{{\hat{C}}}_{{\left[ {\mu \nu } \right.}}}^{{\left[ \alpha \right.}}{{{\hat{C}}}_{{\rho \left| s \right|}}}^{{\left. \beta \right]}}{{A}_{{\left. \sigma \right]}}}^{s}\end{array}](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-44dd55d1d565a93396ba04cf4658051f_l3.png)
Now the definition of F-theory can be summarized as a 12-D lift of Type-IIB with varying axio-dilaton and a 7-brane geometric backreaction where the ![]() duality U-action yields a monodromy-group representation induced by elliptic toric fibration that admits a duality with M-theory via a KK-reduction. The
duality U-action yields a monodromy-group representation induced by elliptic toric fibration that admits a duality with M-theory via a KK-reduction. The ![]() -EFT diffeomorphism group action has two Sen singularity solutions, one corresponding to F-theory, the other to 11-D SUGRA. Hence, such a 12-D field theory dimensionally reduces in the Witten-Vafa limit, to 11-D SUGRA and 10-D Type-IIB theories.
-EFT diffeomorphism group action has two Sen singularity solutions, one corresponding to F-theory, the other to 11-D SUGRA. Hence, such a 12-D field theory dimensionally reduces in the Witten-Vafa limit, to 11-D SUGRA and 10-D Type-IIB theories.
Since the D7-brane backreaction is central to F-theory, let’s study it under the ![]() -EFT/11-D/Type-IIB duality. Solutions to the D7-brane worldvolume action in Type-IIB SUGRA possess non-trivial axio-dilaton and metric. Let a D7-brane extend along the six-dimensions of the compactifying manifold in the
-EFT/11-D/Type-IIB duality. Solutions to the D7-brane worldvolume action in Type-IIB SUGRA possess non-trivial axio-dilaton and metric. Let a D7-brane extend along the six-dimensions of the compactifying manifold in the ![]() and
and ![]() directions, and we represent the time-direction transverse to the brane in polar coordinates
directions, and we represent the time-direction transverse to the brane in polar coordinates ![]() . Thus, the harmonic functional of the D7-brane is
. Thus, the harmonic functional of the D7-brane is ![]() and the solutions to our system are given by:
and the solutions to our system are given by:
![]()
![]()
![]()
![]()
Holomorphically circling the transverse dimension yields, by monodromy group action on ![]() ,
, ![]() :
:

The D7-brane is derived via Type-IIB sectional dimensional reduction under the elliptic fibrational structure underlying the monodromy action on the toric geometry:
![]()
![]()
![]()
On the M-section, we have a monopole-smearing solution:
![Rendered by QuickLaTeX.com \displaystyle \begin{array}{l}\text{d}s_{{\left( {11} \right)}}^{2}=-\text{d}{{t}^{2}}+\text{d}\overrightarrow{{x_{{\left( 6 \right)}}^{2}}}+H\left( {\text{d}{{r}^{2}}+{{r}^{2}}\text{d}{{\theta }^{2}}+{{{\left( {\text{d}{{y}^{1}}} \right)}}^{2}}} \right)\\+{{H}^{{-1}}}{{\left[ {\text{d}{{y}^{2}}+h\theta \text{d}{{y}^{1}}} \right]}^{2}}\end{array}](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-68f3812e3228f6d88ad2c388035a7440_l3.png)
hence, by the M/Type-IIB duality, the smearing relates the first Chern class of the Witten-Vafa fibration to the co-dimension 2 monodromy hypercharges. By modularity, the D7-brane hyperdoublet and the D3-brane multiplet are smeared monopoles that pq-7-branes in Type-IIB with p-cycles and q-cycles holomorphically wrap. The metric of a pq-Type-IIB theory is given as:
![Rendered by QuickLaTeX.com \displaystyle \begin{array}{c}\text{d}s_{{\left( 3 \right)}}^{2}=\frac{{{{H}^{{-1}}}}}{{{{p}^{2}}+{{q}^{2}}}}\left\{ {\left[ {{{p}^{2}}{{H}^{2}}+{{{\left( {ph\theta -q} \right)}}^{2}}} \right]{{{\left( {\text{d}{{y}^{1}}} \right)}}^{2}}} \right.\\+\left[ {{{{\left( {p+qh\theta } \right)}}^{2}}+{{q}^{2}}{{H}^{2}}} \right]{{\left( {\text{d}{{y}^{2}}} \right)}^{2}}\\-2\left[ {\left( {{{p}^{2}}-{{q}^{2}}} \right)h\theta +pq\left( {K-1} \right)} \right]\left. {\text{d}{{y}^{1}}\text{d}{{y}^{2}}} \right\}+{{\left( {{{\text{d}}^{s}}} \right)}^{2}}\end{array}](https://www.georgeshiber.com/wp-content/ql-cache/quicklatex.com-466888942f19045d9c7c365a90a61ae6_l3.png)
Thus, the exceptionality property determines two holomorphic functions ![]() and
and ![]() where the toric modulus takes the form:
where the toric modulus takes the form:
![]()
with the elliptic invariant Jacobian. The polynomial roots give rise to Type-IIB singularities localizing the 7-branes. Hence we get:
![]()
and for the M-section, we have:
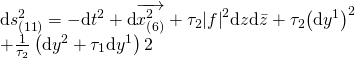
Both maintain the elliptic fibrational base singularities under which the action of the M/Type-IIB duality symmetry gives rise to generalized Klebanov-Witten quiver gauge theories that yield the YM gauge theories of the SM. Exceptionality hence eliminates the need to go to the full-blown 12-D F-theory and thus eliminates both, Betti-truncations and the KK-conical blow-up sector that would have to be super-Higgsed away upon dimensional reduction to 11-D SUGRA. This is phenomenologically important as we shall see in upcoming posts.
