SuSy, Kähler Supermultiplets, and the Degeneracy Problem: D3-Brane Green–Schwarzing

By George Shiber Monday, June 8, 2015Friday, October 26, 2018 In Grand Unification Physics, M Theory, Mathematical Physics, Philosophy Of Mathematics, Philosophy Of Physics, Philosophy Of Science, Super String Theory, Supersymmetry Cauchy principal value, D3-branes

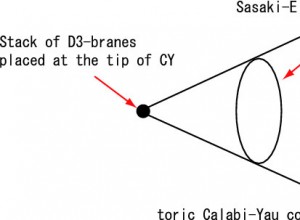
The whole thing that makes a mathematician’s life worthwhile is that he gets the grudging admiration of three or four colleagues. ~ Donald Knuth!

It is disturbing for a superstring/M-theorist to still have to come across the accusation that supersymmetry implies that all members of a Kähler supermultiplet have the same mass. That would pose serious and deep problems for SuSy: first, by SR‘s $e = m{c^2}$ , the quantum vacua of the supergravity theory would have infinite quantum fluctuational field frequency. And more importantly, its Schwarz-Green action:

${S_{SG}} = \int {{d^4}} x{\varepsilon ^{\mu \nu \rho \sigma }}\left\{ {{R_{\mu \nu }}{{(M)}^{ab}}{R_{\rho \sigma }}{{(M)}^{cd}}{\varepsilon _{abcd}} + {R_{\mu \nu }}({Q^a}){R_{\rho \sigma }}({Q^\beta }){{\left( {{\gamma _5} \cdot C} \right)}_{\alpha \rho }}} \right\}$

with ${M_A}$ the Orthosymplectic group $Osp(N/M)$ generators, and

$\left\{ {\begin{array}{*{20}{c}}{{Q^\alpha } \equiv {{\left( {2{p^ + }} \right)}^{1/2}}{S_{SG}}}\\{{Q^\beta } \equiv {{\left( {{p^ + }} \right)}^{ - 1/2}}\gamma _{{a^ \circ }a}^i\sum\limits_{n = - \infty }^\infty {{S_{SG}}\alpha _n^i} }\end{array}} \right.$

would only be integrable over spacetimes with degenerate topologies, and the all too important Schwarz-Green action in 10-dimension,

$\begin{array}{c}S_{sg}^{10} = \frac{{ - 1}}{{4{\alpha ^ * }\pi }}\int {d\sigma d\tau \left\{ {\sqrt g } \right.} {g^{\alpha \beta }}{\prod _\alpha } \cdot {\prod _\beta } + {{\rm Z}_i}{\varepsilon ^{\alpha \beta }}\partial {X^\mu }\left( {{{\overline \theta }^1}{\Gamma _\mu }{{\overline \partial }_\beta }{\theta ^1}{\theta ^2}{\Gamma _\mu }{\partial _\beta }{\theta ^2}} \right)\\ - 2{\varepsilon ^{\alpha \beta }}\overline {\theta \,} {\Gamma ^\mu }{\partial _\alpha }{\theta ^1}\overline {{\theta ^2}} \Gamma {\partial _\beta }\,\left. {{\theta ^2}} \right\}\end{array}$

would not be solvable with respect to any manifold that is isomorphic to a Minkowskian one, where

$\left\{ {\begin{array}{*{20}{c}}{\delta {\theta ^A} = {\varepsilon ^A}}\\{\delta {X^\mu } = i\overline \varepsilon A}\\{{\Gamma ^\mu }{\theta ^A} = 0}\end{array}} \right.$

and $S_{sg}^{10}$ is locally supersymmetric due to the crucial Fierz transformational identity

${\Gamma _\mu }{\psi _{\left[ {1\overline {{\psi _2}} {\Gamma ^\mu }{\psi _3}} \right]}} = 0$

To show that SuSy does not imply massive degeneracy of Kähler supermultiplets, a few more mathematical set-up is required, while mentioning a few more concerns to be addressed. One takes advantage of the fact that the energy-momentum operator can be decomposed into normal nodes

${P_n} = \oint {\frac{{dz}}{{2\pi i}}\,} z_\infty ^{n + 1}T(z)$

where

$T(z) = \sum\limits_{n = - \infty }^\infty {{z^{ - n - 2}}} {L_n}$

and the following crucial relation holds:

$\begin{array}{c}\left[ {{P_{n,}}\,{L_n}} \right] = \oint {\frac{{dw}}{{2\pi i}}} \,{w^{n + 1}}\oint {\frac{{dz}}{{2\pi i}}} \,{z^{n + 1}} \times \\\left[ {\frac{{\frac{1}{2}C}}{{{{\left( {z - w} \right)}^4}}} + \frac{2}{{{{\left( {z - w} \right)}^2}}}T(z) + \frac{1}{{\left( {z - w} \right)}}{\partial _w}T(z)} \right] + \\\oint {\frac{{dw}}{{d\pi i}}} \,{w^{n + 1}}\left\{ {\left( {n + 1} \right)wT(z) + {w^{n + 1}}{\partial _w}T(z) + \frac{{\frac{1}{2}C}}{{3!}}{w^{n - 2}}\left( {n + 1} \right)n\left( {n - i} \right)} \right\}\\ = \oint {\frac{{dw}}{{d\pi i}}} \left\{ {\left( {2n + 2} \right){w^{n + m + 1}}T(z) + \frac{C}{{12}}n\left( {n + 1} \right)\left( {n - 1} \right)w} \right\}\end{array}$

Another deep problem for SuSy would be that, by the Fierz transformational equation, SuSy would lose its Poincaré invariance, and hence the SuSy generators and the Poincaré group generators would not be in integral convolution, which is a contradiction. Thirdly, it would be mathematically impossible, in the Hilbert super-space setting, for the creation-annihilation operator to dynamically create superpartners, and hence, the Sboson would not exist, contra the fact that superstring theory entails their existence: so, the catastrophe would be – no Sbosons, no supergravity. Moreover, to magnify the severity of the problem, the Gibbs probability density, with ${\varphi _t}(x)$ being the gauge configuration field of SuGra at ‘t’, will ‘go’ to infinity:

${\rho _G}\left[ {{\varphi _t}} \right] = \exp \left[ { - \frac{1}{\hbar }\int {\frac{{{d^3}k}}{{{{\left( {2\pi } \right)}^3}}}\widetilde {{\varphi _t}}{{(k)}^ * }\sqrt {{{\left| k \right|}^2} + {m^2}} \widetilde {{\varphi _t}}(k)} } \right] \to \infty$

and thus is a clear violation of Lorentz invariance. So, with all that in hand, let me disprove the claim that SuSy has a Kähler supermultiplet mass equality degeneracy problem.

Let ${\psi _{kd}}$ be a Kähler-Dirac 4-component complex spinor fermionic field: it clearly, by Kac-Moody Laurent polynomial expansion, obeys the following equation of motion:

$\left( {\not p - m\varepsilon ({p_0})} \right){\psi _{kd}}(p) = 0$

which is solvable if and only if the Klein-Fock-Gordon equation is, which is as desired. The Kähler-Dirac theory actually represents the ‘Kac-Moody’ square root of the Klein-Fock-Gordon equation: hence, the Kähler-Dirac theory is central because the Kähler equation of motion is non-local in positional phase space

$i\not \partial {\psi _{kd}}(t,x) = - \frac{{im}}{\pi }{p_k}\int {d{t^ * }} \frac{1}{{t - {t^ * }}}{\psi _{kd}}({t^ * },x)$

where $p$ is the Cauchy principal value. It is then clear that the above KD equation is Lorentz invariant on-shell! Now, when a Kähler-Dirac mass term is coupled with a Dirac mass term, CPT is violated: to see this, consider the Kähler free Lagrangian

$\int {{d^3}} x\overline {{\psi _{kd}}} \left( {i\not \partial - {m_d}} \right){\psi _{kd}} + \frac{{i{m_h}}}{\pi }\int {\frac{{{d^3}xd{t^ * }}}{{t - {t^ * }}}} \left( {\overline {{\psi _{kd}}} (t)\psi ({t^ * }) - \overline {{\psi _{kd}}} (t)\psi ({t^ * })} \right)$

The CPT conjugate spinor have eingenvalues that are mass-squared

${\left( {{m_d} \pm {m_h}} \right)^2}$

We must extend the Kähler-Dirac theory to a hyper-super-multiplet of superfields which induce the N=2 SuSy algebra representation. We first note that, with $i = 1,2$ , the generator

$Q_i^\beta = {(p_i^ + )^{ - 1/2}}\gamma _{{a^ \circ }a}^i\sum\limits_{n = - \infty }^\infty {S_{SG}^{10}} \alpha _n^i$

are the metaplectic Majorana spinor supercharges which form a super-doublet under $SU(2)$ R symmetry of N=2 SuSy. Letting ${\Psi _j}(x)$ be a complex scalar doublet field, with $j$ raised/lowered with the Levi-Civita symbol

${\varepsilon _{ij}} = {\varepsilon ^{ij}}$

and $\vartheta (x)$ a 4-component complex fermionic field: we then have the critical relation:

$\left[ {Q_i^\beta ,{\Psi _j}(x)} \right] = - i\sqrt {2\pi i} \delta _j^i\,\vartheta (x)$

Now, note that from the above relation, together with the D3-brane ‘Green-Schwarz-ing’ Lagrangian-identity:

${L_{GS}} = \left[ {\int {{d^3}x\overline {{\psi _{kd}}} (i\not \partial - {m_d}){\psi _{kd}} + \frac{{i{m_h}}}{\pi }\int {\frac{{{d^3}xd{t^ * }}}{{t - {t^ * }}}\left( {\overline {{\psi _{kd}}} (t)\psi ({t^ * })} \right) - \overline \psi ({t^ * })\psi (t)} } } \right]_{D3 - {\rm{BRANE}}}^{S_{SG}^{10}}$

one obtains the Kähler-Dirac supersymmetry via super-auxiliary D3-brane world-volume fields:

${\Sigma _i}(x) = i{m_d}{\Psi _t}$

satisfying

${\Sigma _i}(x) = - \,i{m_d}{\Psi _t}(x) - \frac{{{m_h}}}{\pi }\int {\frac{{d{t^ * }}}{{t - {t^ * }}}} {\Psi _i}({t^ * },x)$

and solving it gives us

$S = \int {dt\left( {{L_{{\rm{Kinetic}}}} + {L_n} + {P_n} + T(z)} \right)}$

and all 4 parts:

$\left\{ {\begin{array}{*{20}{c}}{{L_{{\rm{Kinetic}}}}}\\{{L_n}}\\{{P_n} = \oint {\frac{{dz}}{{2\pi i}}{z^{n + 1}}\sum\limits_{n = - \infty }^\infty {{z^{ - n - 2}}{L_n}} } }\\{T(z)}\end{array}} \right.$

are N=2 SuSy invariants, as required to solve the ‘mass’ problem. To finish the proof, we note that the classic Noether method does not lead to current conservation: one must introduce a supersymmetric conserved bosonic current $J_B^j(x)$ belonging to a supermultiplet of the Noether conserved current. One then has, by Fierz transformation:

$\begin{array}{c}\frac{{J_B^j(x)}}{{\sqrt 2 }} = {\gamma ^\mu }{\vartheta _j}{\Psi ^i} + {\delta ^{\mu 0}}\frac{{{m_n}}}{\pi }\int_{ - t}^t {d{t^ * }} \int {\frac{{d{t^ * }}}{{{t^ * } - t_i^ * }}} \left[ {\vartheta (t_i^ * ){\Psi _i}} \right.({t^ * }) + \\{\gamma ^\nu }\vartheta (t_i^ * ){\partial _\nu }\Psi _i^ * ({t^ * }) + \left. {\left( {{t^ * } \leftrightarrow t_i^ * } \right)} \right]\end{array}$

and, by 2nd-quantization, it follows that ${\Psi _B}(x)$ , with its conjugate ${\prod ^j}(x)$ , are given by:

$\begin{array}{c}\left[ {\Psi _B^i(x){\prod ^j}({x^j})} \right] = \frac{i}{2}\delta _i^j\int {\frac{{{d^3}{P_n}}}{{{{(2\pi )}^3}}}} \cdot \\\exp \left( { - iw + (t - {t^ * }) + i{P_n}(x - {x^ * })} \right) + \\\exp \left( {iw - (t - {t^ * })} \right) - \left( {i{P_n}(x - {x^ * })} \right)\end{array}$

Thus, the Planck-time commutator of $\Psi _B^i(x)$ , with its conjugate ${\prod ^i}(x)$

is:

$\Psi _B^i = \int {\frac{{{d^3}{P_n}}}{{{{(2\pi )}^3}}}} \left( {\frac{{{a_{{P_n}}}}}{{\sqrt {2w + } ...}}{e^{ - wt + i{P_n}}} + \frac{{{P_n}}}{{\sqrt {2w - } ...}}{e^{iwt - i{P_n}t}}\left( {i{P_n} \cdot x} \right)} \right)$

where

$w = \sqrt {P_n^2 + m_d^2}$

and we thus arrive at the Sbosonic super-Hamiltonian

${H_{Sb}} = \int {{d^3}} x\left[ {w + {a^\dagger }({P_n})a(P_n^i) + w - {b^\dagger }({P_n})b(P_n^i)} \right]$

and the Sfermionic one

${H_{Sf}} = \int {{d^3}} x\left[ {w + \widetilde a(P_n^i)\widetilde a({P_n}) + w - {b^\dagger }({P_n})\widetilde b(P_n^i)} \right]$

The key now to solving the purported ‘SuSy’-mass supermultiplet degeneracy problem (given all our machinery above), is to realize that the following identities are true

$\left\{ {\begin{array}{*{20}{c}}{\delta {H_{Sb}} = {{\left\langle {\int {{S_{SG}}({P_n})} } \right\rangle }_{3D - {\rm{BRANE}}}} = 0}\\{\delta {H_{Sf}} = {{\left\langle {\int {{S_{SG}}({L_n})} } \right\rangle }_{3D - {\rm{BRANE}}}} = 0}\end{array}} \right.$

So by D3-branes Green–Schwarz analysis, one solves the ‘mass-degeneracy’ problem, and thus SuSy does not entail that all members of a Kähler supermultiplet have equal mass.

I might add: mathematics is not only the king of the ‘sciences’, but is the most ethical field of inquiry, for it is directly responsible for progress in all, yet gets no favors back in kind at all!